Giải bất phương trình x3−4x<0
cần gấp
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


`cosx.tan(\pi+x)+cos(-x)-sin(\pi/2-x)+sin(\pi-x)`
`=cosx.tanx+cosx-cosx+sinx`
`=cosx . [ sinx]/[cosx]+sinx`
`=sinx+sinx`
`=2sinx`
tôi chọn toán nó cứ bảo vui lòng chọn môn nên thấy hóa học thì kệ mẹ nó đi nha

Điều kiện x + 1 khác 0 => x khác -1
Có 2 trường hợp thỏa mãn yêu cầu
Trường hợp 1: x + 1 > 1 => x > 0
Trường hợp 2: x+ 1 < 0 => x < -1
Kết luận x > 0 hoặc x < -1

Ta có \(\dfrac{\sin\alpha}{\cos\alpha}=\tan\alpha=2\Rightarrow\sin\alpha=2\cos\alpha\)
Lại có \(\sin^2\alpha+\cos^2\alpha=1\Rightarrow4\cos^2\alpha+\cos^2\alpha=1\)\(\Rightarrow5\cos^2\alpha=1\Rightarrow\cos^2\alpha=\dfrac{1}{5}\Rightarrow\cos\alpha=\dfrac{\sqrt{5}}{5}\)
\(\Rightarrow\sin\alpha=2\cos\alpha=\dfrac{2\sqrt{5}}{5}\)
Ta có:
\(2=tana=\dfrac{sina}{cosa}\Leftrightarrow sina=2cosa\)
\(sin^2a+cos^2a=1\Rightarrow4cos^2a+cos^2a=1\Leftrightarrow cos^2a=\dfrac{1}{5}\Leftrightarrow cosa=\dfrac{\pm\sqrt{5}}{5}\)
- \(cosa=\dfrac{\sqrt{5}}{5}\Rightarrow sina=\dfrac{2\sqrt{5}}{5}\).
- \(cosa=\dfrac{-\sqrt{5}}{5}\Rightarrow sina=\dfrac{-2\sqrt{5}}{5}\).


a) Dễ thấy tứ giác BDHF có \(\widehat{BDH}+\widehat{BFH}=90^o+90^o=180^o\)
\(\Rightarrow\) Tứ giác BDHF nội tiếp (dhnb)
Tứ giác BCEF có \(\widehat{BEC}=\widehat{BFC}\left(=90^o\right)\)
\(\Rightarrow\) Tứ giác BCEF nội tiếp (dhnb)
b) Tứ giác BDHF nội tiếp (cmt) \(\Rightarrow\widehat{DFH}=\widehat{DBH}\) hay \(\widehat{DFC}=\widehat{EBC}\)
Tứ giác BCEF nội tiếp (cmt) \(\Rightarrow\widehat{EFC}=\widehat{EBC}\)
Từ đó ta có \(\widehat{DFC}=\widehat{EFC}\left(=\widehat{EBC}\right)\)
\(\Rightarrow\) FC là tia phân giác \(\widehat{EFD}\) (đpcm)
c) Ta có \(\widehat{DFC}=\widehat{EFC}\) (cmt) \(\Rightarrow90^o-\widehat{DFC}=90^o-\widehat{EFC}\)\(\Rightarrow\widehat{AFE}=\widehat{BFD}\)
Mà \(\widehat{AFE}=\widehat{BFM}\) (2 góc đối đỉnh) \(\Rightarrow\widehat{BFD}=\widehat{BFM}\)
\(\Rightarrow\) FB là tia phân giác của \(\widehat{DFM}\)
Theo tính chất đường phân giác trong tam giác DFM, ta có \(\dfrac{FD}{FM}=\dfrac{BD}{BM}\)
Lại có FC là tia phân giác \(\widehat{EFD}\) (cmt), theo tính chất đường phân giác của góc ngoài của tam giác, ta có \(\dfrac{FD}{FM}=\dfrac{CD}{CM}\)
Do đó, ta có \(\dfrac{BD}{BM}=\dfrac{CD}{CM}\)\(\Rightarrow\dfrac{BD}{CD}=\dfrac{BM}{CM}\) (1)
\(\Delta MAC\) có \(BI//AC\left(gt\right)\) nên \(\dfrac{BI}{AC}=\dfrac{BM}{CM}\) (2) (định lý Ta-lét)
\(\Delta ACD\) có \(BK//AC\left(gt\right)\) nên \(\dfrac{BK}{AC}=\dfrac{BD}{CD}\) (3) (hệ quả định lý Ta-lét)
Từ (1), (2), (3) \(\Rightarrow\dfrac{BI}{AC}=\dfrac{BK}{AC}\Rightarrow BI=BK\)
\(\Rightarrow\) B là trung điểm IK \(\Rightarrow\) HB là trung tuyến của \(\Delta HIK\)
Mặt khác AC//IK (gt), lại có \(BE\perp AC\) nên \(BE\perp IK\), từ đó suy ra HB là đường cao của \(\Delta HIK\)
\(\Delta HIK\) có HB vừa là đường cao vừa là trung tuyến \(\Rightarrow\Delta HIK\) cân tại H (đpcm)
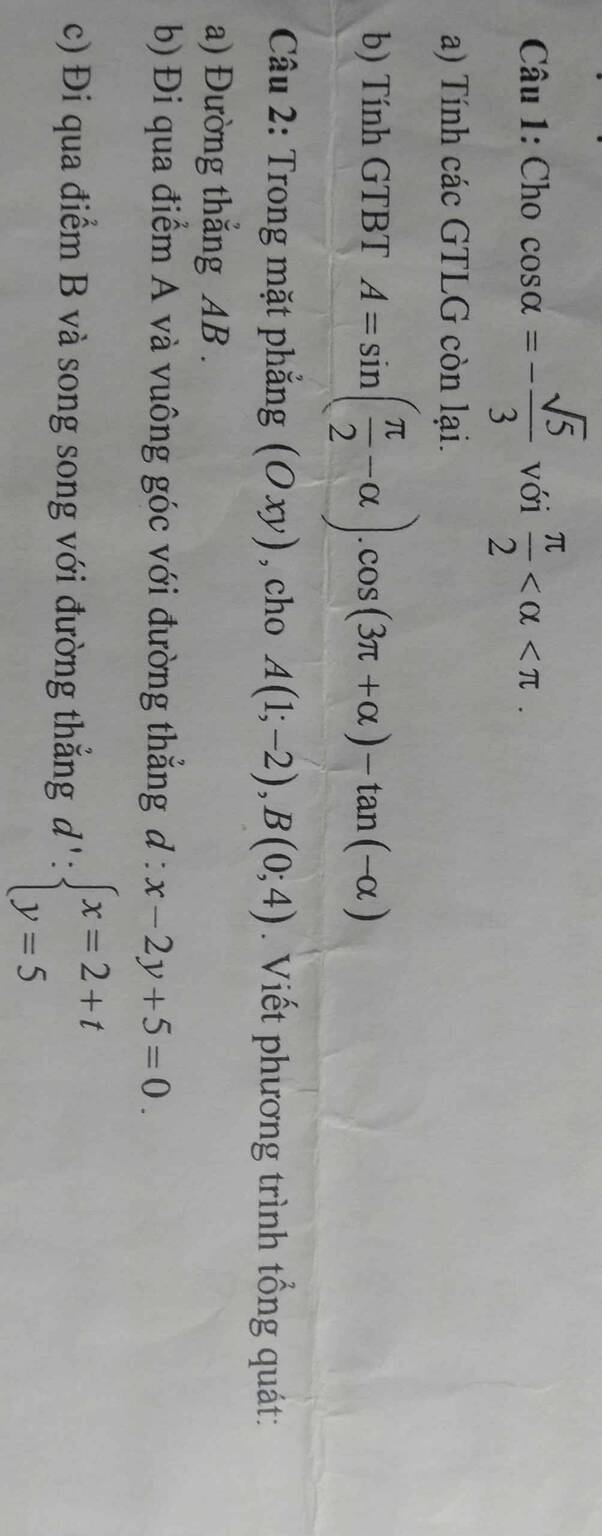
Lời giải:
$x^3-4x<0$
$\Leftrightarrow x(x^2-4)<0$
Xét 2 TH:
TH1: \(\left\{\begin{matrix} x<0\\ x^2-4>0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x<0\\ (x-2)(x+2)>0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x<0\\ \text{x>2 hoặc x< -2}\end{matrix}\right.\)
\(\Leftrightarrow x< -2\)
TH2: \(\left\{\begin{matrix} x>0\\ x^2-4<0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x>0\\ (x-2)(x+2)<0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x>0\\ -2< x< 2\end{matrix}\right.\)
\(\Rightarrow 0< x< 2\)
Vậy tập nghiệm của BPT là $(0;2)\cup (-\infty; -2)$
\(x^3-4x< 0\Leftrightarrow x\left(x^2-4\right)< 0\)
Có 2 trường hợp xảy ra.
TH1: \(\left\{{}\begin{matrix}x>0\\x^2-4< 0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x>0\\x^2< 4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x>0\\-2< x< 2\end{matrix}\right.\Leftrightarrow0< x< 2\)
TH2: \(\left\{{}\begin{matrix}x< 0\\x^2-4>0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x< 0\\x^2>4\end{matrix}\right.\) (*)
Ta có \(x^2>4\Leftrightarrow\left[{}\begin{matrix}x>2\\x< -2\end{matrix}\right.\), vậy (*) \(\Leftrightarrow x< -2\)
Vậy tập nghiệm của BPT đã cho là \(x\) sao cho \(0< x< 2\) hoặc \(x< -2\)