So sánh 11/10 và 10/11
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(A=\dfrac{10^{12}+6}{10^{12}-11}\)
\(\Rightarrow A=\dfrac{10^{12}-11+17}{10^{12}-11}\)
\(\Rightarrow A=\dfrac{10^{12}-11}{10^{12}-11}+\dfrac{17}{10^{12}-11}\)
\(\Rightarrow A=1-\dfrac{17}{10^{12}-11}\)
\(B=\dfrac{10^{11}+5}{10^{11}-12}\)
\(\Rightarrow B=\dfrac{10^{11}-12+17}{10^{11}-12}\)
\(\Rightarrow B=\dfrac{10^{11}-12}{10^{11}-12}+\dfrac{17}{10^{11}-12}\)
\(\Rightarrow B=1-\dfrac{17}{10^{11}-12}\)
Vậy ta cần so sánh \(1-\dfrac{17}{10^{12}-11}\) và \(1-\dfrac{17}{10^{11}-12}\)
Ta thấy \(\left(10^{12}-11\right)>\left(10^{11}-12\right)\) và 2 phân số trên cùng tử số 17 nên \(\dfrac{17}{10^{12}-11}< \dfrac{17}{10^{11}-12}\)
Vậy \(1-\dfrac{17}{10^{12}-11}>1-\dfrac{17}{10^{11}-12}\) hay \(A>B\)

Ta có :
\(A=\dfrac{10^{11}-1}{10^{12}-1}< 1\)
\(\Leftrightarrow A< \dfrac{10^{11}-1+11}{10^{12}-1+11}=\dfrac{10^{11}+10}{10^{12}+10}=\dfrac{10\left(10^{10}+1\right)}{10\left(10^{11}+1\right)}=\dfrac{10^{10}+1}{10^{11}+1}=B\)
Vậy \(\dfrac{10^{11}-1}{10^{12}-1}< \dfrac{10^{10}+1}{10^{11}+1}\)
Vậy...

\(A=\dfrac{10^{11}+1}{10^{12}-1}\)
\(\Rightarrow10A=\dfrac{10^{11}+1}{10^{12}-1}.10\)
\(\Rightarrow10A=\dfrac{10\left(10^{11}+1\right)}{10^{12}-1}\)
\(\Rightarrow10A=\dfrac{10^{12}-10}{10^{12}-1}\)
\(B=\dfrac{10^{10}+1}{10^{11}+1}\)
\(\Rightarrow10B=\dfrac{10^{10}+1}{10^{11}+1}.10\)
\(\Rightarrow10B=\dfrac{\left(10^{10}+1\right).10}{10^{11}+1}\)
\(\Rightarrow10B=\dfrac{10^{11}+10}{10^{11}+1}\)
Ta thấy:
\(10^{12}-1>10^{12}-10>0\Rightarrow10A< 1\)
\(0< 10^{11}+1< 10^{11}+10\Rightarrow10B>1\)
Mà \(10A< 1;10B>1\)
\(\Rightarrow B>A\).

a)\(\dfrac{19}{10}>\dfrac{10}{11}\)
b)\(\dfrac{11}{10}=\dfrac{12}{11}\)
c)\(\dfrac{9}{10}< \dfrac{10}{11}\)


B/A= [(10^10 + 1)/(10^11 + 1)]/[(10^11 - 1)/(10^12 - 1)]
= [(10^12 - 1).(10^10 + 1)]/[(10^11 - 1).(10^11 + 1)]
= [(10^22 - 1) + (10^12 - 10^10) ]/((10^22 - 1)
= 1 + (10^12 - 10^10)/(10^22 - 1) > 1
=> B > A

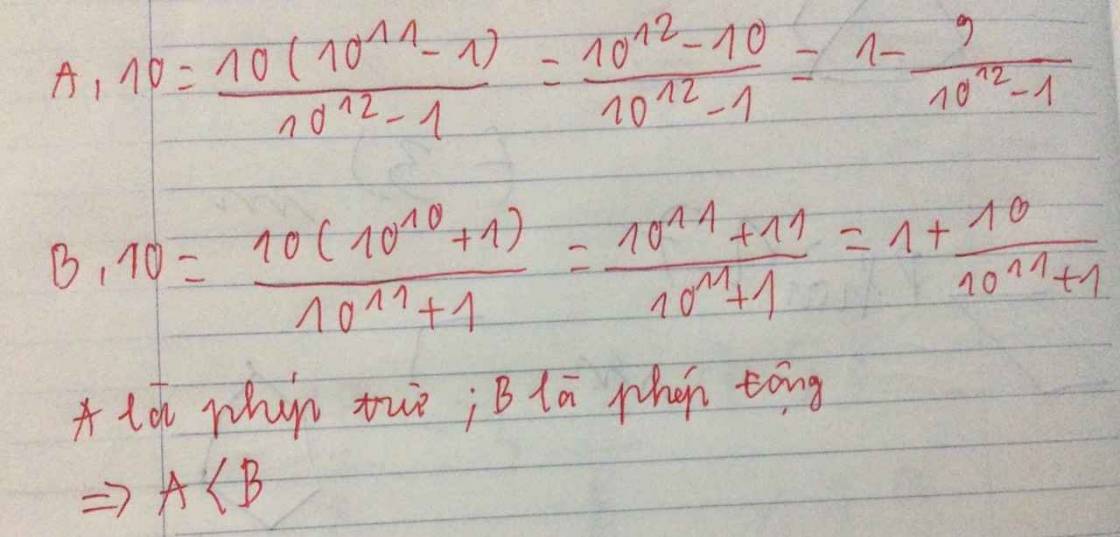
`MSC:110`
`11/10 =(11xx11)/(10xx11)= 121/110`
`10/11=(10xx10)/(11x10)=100/110`
`121>100=>11/10>10/11`
>