Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Bài 1: (Sgk/36):
a. \(\dfrac{5y}{7}\)=\(\dfrac{20xy}{28x}\) vì
5y . 28x = 140xy
7 . 20xy = 140xy
=> 5y . 28x = 7 . 20xy
Vậy \(\dfrac{5y}{7}\)=\(\dfrac{20xy}{28x}\)
b. \(\dfrac{3x\left(x+5\right)}{2\left(x+5\right)}\)=\(\dfrac{3x}{2}\) vì
3x . 2(x+5) = 6x2+30x
2 . 3x(x+5) = 6x2+30x
=> 3x . 2(x+5) = 2 . 3x(x+5)
Vậy \(\dfrac{3x\left(x+5\right)}{2\left(x+5\right)}\)=\(\dfrac{3x}{2}\)
c. \(\dfrac{x+2}{x-1}\)=\(\dfrac{\left(x+2\right)\left(x+1\right)}{x^2-1}\) vì
(x+2) (x2-1) = (x+2) (x-1) (x-1)
=> (x+2) (x2-1) = (x-1) (x+2) (x+1)
Vậy \(\dfrac{x+2}{x-1}\)=\(\dfrac{\left(x+2\right)\left(x+1\right)}{x^2-1}\)
d. \(\dfrac{x^2-x-2}{x+1}\)=\(\dfrac{x^2-3x+2}{x-1}\)
(x-1) (x2-x-2) = x3-2x2-x+2
(x+1) (x2-3x+2) = x3-2x2-x+2
=> (x-1) (x2-x-2) = (x2-3x+2) (x+1)
Vậy \(\dfrac{x^2-x-2}{x+1}\)=\(\dfrac{x^2-3x+2}{x-1}\)

Câu 1 :
a) Rút gọn P :
\(P=\dfrac{x+1}{3x-x^2}:\left(\dfrac{3+x}{3-x}-\dfrac{3-x}{3+x}-\dfrac{12x^2}{x^2-9}\right)\)
\(P=\dfrac{x+1}{x\left(3-x\right)}:\left[\dfrac{\left(3+x\right)^2}{\left(3-x\right)\left(3+x\right)}-\dfrac{\left(3-x\right)^2}{\left(3-x\right)\left(3+x\right)}-\dfrac{12x^2}{\left(3-x\right)\left(3+x\right)}\right]\)
\(P=\dfrac{x+1}{x\left(3-x\right)}:\left(\dfrac{9+6x+x^2-9+6x-x^2-12x^2}{\left(3-x\right)\left(3+x\right)}\right)\)
\(P=\dfrac{x+1}{x\left(3-x\right)}:\dfrac{12x-12x^2}{\left(3-x\right)\left(x+3\right)}\)
\(P=\dfrac{x+1}{x\left(3-x\right)}.\dfrac{\left(3-x\right)\left(x+3\right)}{12x\left(1-x\right)}\)
\(P=\dfrac{\left(x+1\right)\left(x+3\right)}{12x^2\left(1-x\right)}\)

a)
Đặt
\(\sqrt{1+x}=a; \sqrt{1-x}=b\Rightarrow \left\{\begin{matrix} ab=\sqrt{(1+x)(1-x)}=\sqrt{1-x^2}\\ a\geq b\\ a^2+b^2=2\end{matrix}\right.\)
Khi đó:
\(A=\frac{\sqrt{1-\sqrt{1-x^2}}(\sqrt{(1+x)^3}+\sqrt{(1-x)^3})}{2-\sqrt{1-x^2}}\)
\(=\frac{\sqrt{\frac{a^2+b^2}{2}-ab}(a^3+b^3)}{a^2+b^2-ab}=\frac{\sqrt{\frac{a^2+b^2-2ab}{2}}(a+b)(a^2-ab+b^2)}{a^2+b^2-ab}\)
\(=\sqrt{\frac{a^2-2ab+b^2}{2}}(a+b)=\sqrt{\frac{(a-b)^2}{2}}(a+b)=\frac{1}{\sqrt{2}}|a-b|(a+b)\)
\(=\frac{1}{\sqrt{2}}(a-b)(a+b)=\frac{1}{\sqrt{2}}(a^2-b^2)=\frac{1}{\sqrt{2}}[(1+x)-(1-x)]=\sqrt{2}x\)
Sửa đề: \(\frac{25}{(x+z)^2}=\frac{16}{(z-y)(2x+y+z)}\)
Ta có:
Áp dụng tính chất dãy tỉ số bằng nhau thì:
\(k=\frac{a}{x+y}=\frac{5}{x+z}=\frac{a+5}{2x+y+z}=\frac{5-a}{z-y}\) ($k$ là một số biểu thị giá trị chung)
Khi đó:
\(\frac{16}{(z-y)(2x+y+z)}=\frac{25}{(x+z)^2}=(\frac{5}{x+z})^2=k^2\)
Mà: \(k^2=\frac{a+5}{2x+y+z}.\frac{5-a}{z-y}=\frac{25-a^2}{(2x+y+z)(z-y)}\)
Do đó: \(\frac{16}{(z-y)(2x+y+z)}=\frac{25-a^2}{(2x+y+z)(z-y)}\Rightarrow 16=25-a^2\)
\(\Rightarrow a^2=9\Rightarrow a=\pm 3\)
Suy ra:
\(Q=\frac{a^6-2a^5+a-2}{a^5+1}=\frac{a^5(a-2)+(a-2)}{a^5+1}=\frac{(a-2)(a^5+1)}{a^5+1}=a-2=\left[\begin{matrix}
1\\
-5\end{matrix}\right.\)

1.
|x-9|=2x+5
x<9; x-9=-2x-5
3x=4=>x=4/3(n)
x≥9; x-9=2x+5=> x=-14(l)
2.a
A=2x-5≥0<=>2x≥5; x≥5/2
1. a) / x - 9 / = 2x + 5
Do : / x - 9 / ≥ 0 ∀x
⇒2x + 5 ≥ 0
⇔ x ≥ \(\dfrac{-5}{2}\)
Bình phương cả hai vế của phương trình , ta được :
( x - 9)2 = ( 2x + 5)2
⇔ ( x - 9)2 - ( 2x + 5)2 = 0
⇔ ( x - 9 - 2x - 5)( x - 9 + 2x + 5) = 0
⇔ ( - x - 14)( 3x - 4) = 0
⇔ x = - 14 ( KTM) hoặc : x = \(\dfrac{4}{3}\) ( TM)
KL....
b) Mạn phép làm luôn , ko chép lại đề :
\(\dfrac{5\left(x+3\right)}{\left(x-3\right)\left(x+3\right)}+\dfrac{4\left(x-3\right)}{\left(x+3\right)\left(x-3\right)}=\dfrac{x-5}{\left(x-3\right)\left(x+3\right)}\) ( x # 3 ; x # - 3)
⇔ 5x + 15 + 4x - 12 = x - 5
⇔ 9x + 3 = x - 5
⇔ 8x = - 8
⇔ x = -1 ( TM)
KL....

Bài 1:
a) \(\dfrac{3x^2-5}{x^2-5x}+\dfrac{5-15x}{5x-25}\)
\(=\dfrac{3x^2-5}{x\left(x-5\right)}+\dfrac{5\left(1-3x\right)}{5\left(x-5\right)}\)
\(=\dfrac{3x^2-5}{x\left(x-5\right)}+\dfrac{1-3x}{x-5}\)
\(=\dfrac{3x^2-5}{x\left(x-5\right)}+\dfrac{x\left(1-3x\right)}{x\left(x-5\right)}\)
\(=\dfrac{3x^2-5+x\left(1-3x\right)}{x\left(x-5\right)}\)
\(=\dfrac{3x^2-5+x-3x^2}{x\left(x-5\right)}\)
\(=\dfrac{-5+x}{x\left(x-5\right)}\)
\(=\dfrac{x-5}{x\left(x-5\right)}\)
\(=\dfrac{1}{x}\)
b) \(\dfrac{4+x^3}{x-3}-\dfrac{2x+2x^2}{x-3}+\dfrac{2x-13}{x-3}\)
\(=\dfrac{\left(4+x^3\right)-\left(2x+2x^2\right)+\left(2x-13\right)}{x-3}\)
\(=\dfrac{4+x^3-2x-2x^2+2x-13}{x-3}\)
\(=\dfrac{x^3-2x^2-9}{x-3}\)
\(=\dfrac{x^3-3x^2+x^2-9}{x-3}\)
\(=\dfrac{x^2\left(x-3\right)+\left(x-3\right)\left(x+3\right)}{x-3}\)
\(=\dfrac{\left(x-3\right)\left(x^2+x+3\right)}{x-3}\)
\(=x^2+x+3\)
c) \(\dfrac{2}{x-5}+\dfrac{x-25}{\left(x+5\right)\left(x-5\right)}\)
\(=\dfrac{2\left(x+5\right)}{\left(x+5\right)\left(x-5\right)}+\dfrac{x-25}{\left(x+5\right)\left(x-5\right)}\)
\(=\dfrac{2\left(x+5\right)+x-25}{\left(x+5\right)\left(x-5\right)}\)
\(=\dfrac{2x+10+x-25}{\left(x+5\right)\left(x-5\right)}\)
\(=\dfrac{3x-15}{\left(x+5\right)\left(x-5\right)}\)
\(=\dfrac{3\left(x-5\right)}{\left(x+5\right)\left(x-5\right)}\)
\(=\dfrac{3}{x+5}\)
d) Đề sai?
Bài 2:
\(A=2\left(x+1\right)+\left(3x+2\right)\left(3x-2\right)-9x^2\)
\(A=2x+2+9x^2-4-9x^2\)
\(A=2x-2\)
\(A=2\left(x-1\right)\)
Thay x = 15 vào A ta được:
\(A=2\left(15-1\right)\)
\(A=2.14=28\)

mk nghỉ bài này đề sai
a) điều kiện : \(x\ne0;x\ne-1;x\ne2\)
ta có : \(A=1+\left(\dfrac{x+1}{x^3+1}-\dfrac{1}{x-x^2-1}+\dfrac{2}{x+1}\right):\dfrac{x^3-2x^2}{x^3-x^2+x}\)
\(\Leftrightarrow A=1+\left(\dfrac{x+1}{\left(x+1\right)\left(x^2-x+1\right)}+\dfrac{1}{x^2-x+1}+\dfrac{2}{x+1}\right):\dfrac{x\left(x-2\right)}{x^2-x+1}\) \(\Leftrightarrow A=1+\left(\dfrac{x+1+x+1+2\left(x^2-x+1\right)}{\left(x+1\right)\left(x^2-x+1\right)}\right):\dfrac{x\left(x-2\right)}{x^2-x+1}\) \(\Leftrightarrow A=1+\left(\dfrac{2x^2+4}{\left(x+1\right)\left(x^2-x+1\right)}\right):\dfrac{x^2-x+1}{x\left(x-2\right)}\) \(\Leftrightarrow A=1+\dfrac{2x^2+4}{x\left(x+1\right)\left(x-2\right)}=\dfrac{2x^2+4+x\left(x+1\right)\left(x-2\right)}{x\left(x+1\right)\left(x-2\right)}\)\(\Leftrightarrow A=\dfrac{x^3+x^2-2x+4}{x\left(x+1\right)\left(x-2\right)}\)
b) ta có : \(\left|x-\dfrac{3}{4}\right|=\dfrac{5}{4}\) \(\Leftrightarrow\left[{}\begin{matrix}x-\dfrac{3}{4}=\dfrac{5}{4}\\x-\dfrac{3}{4}=\dfrac{-5}{4}\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=2\left(L\right)\\x=\dfrac{-1}{2}\end{matrix}\right.\)
thế vào \(A\) ta có : \(A=\dfrac{41}{5}\)
vậy ...............................................................................................................

a)
\(\dfrac{1}{x}-\dfrac{1}{x+1}=\dfrac{x+1-x}{x\left(x+1\right)}=\dfrac{1}{x\left(x+1\right)}\left(đpcm\right)\)
b)
\(\dfrac{1}{x\left(x+1\right)}+\dfrac{1}{\left(x+1\right)\left(x+2\right)}+\dfrac{1}{\left(x+2\right)\left(x+3\right)}+\dfrac{1}{\left(x+3\right)\left(x+4\right)}+\dfrac{1}{\left(x+4\right)\left(x+5\right)}+\dfrac{1}{x+5}\\ =\dfrac{1}{x}-\dfrac{1}{x+1}+\dfrac{1}{x+1}-\dfrac{1}{x+2}+\dfrac{1}{x+2}-\dfrac{1}{x+3}+\dfrac{1}{x+3}-\dfrac{1}{x+4}+\dfrac{1}{x+4}-\dfrac{1}{x+5}+\dfrac{1}{x+5}\\ =\dfrac{1}{x}\)
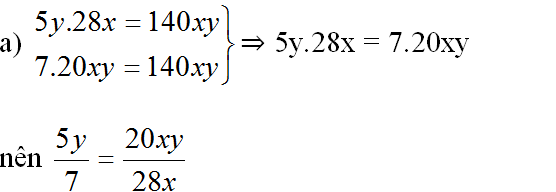
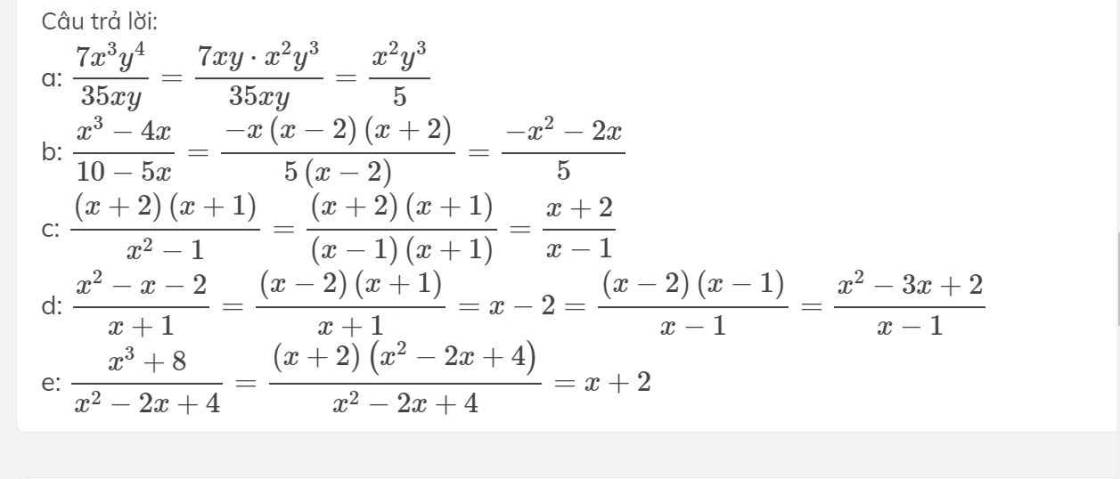
Biến đổi biểu thức A :
\(A=\dfrac{1}{x}+\dfrac{1}{x+5}+\dfrac{x-5}{x\left(x+5\right)}=\dfrac{x+5+x+x-5}{x\left(x+5\right)}=\dfrac{3x}{x\left(x+5\right)}=\dfrac{3}{x+5}=B\)